Quick Review: Arithmetic, Geometric and Harmonic Progressions -

Read Time:2 Minute, 45 Second

In this article, you will learn shortcuts and formulae related to AP, GP and HP.

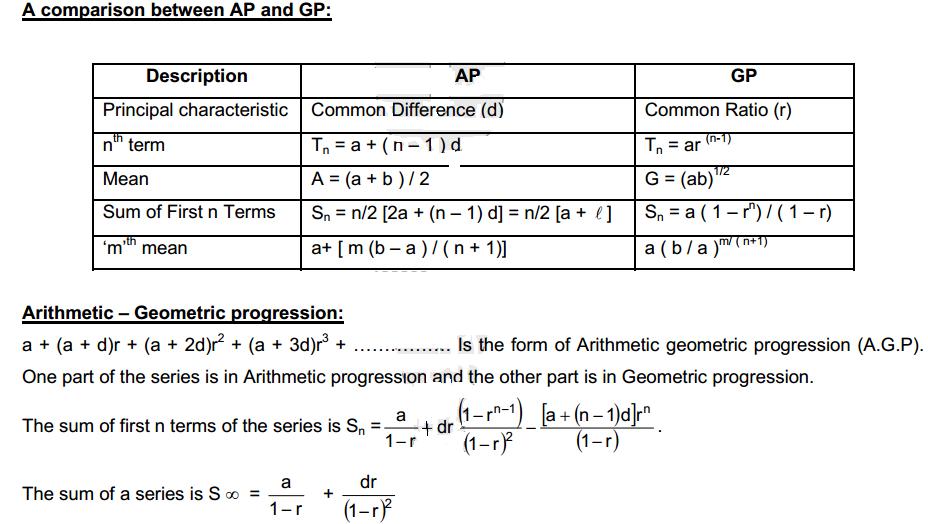
Arithmetic Progression

- An arithmetic progression is a sequence of numbers in which each term is derived from the preceding term by adding or subtracting a fixed number called the common difference “d”
  For example, the sequence 9, 6, 3, 0,-3, …. is an arithmetic progression with -3 as the common difference. The progression -3, 0, 3, 6, 9 is an Arithmetic Progression (AP) with 3 as the common difference.

The general form of an Arithmetic Progression is a, a + d, a + 2d, a + 3d and so on. Thus nth term of an AP series is T_n = a + (n – 1) d, where T_n = n^th term and a = first term. Here d = common difference = T_n – T_n-1.
Sum of first n terms of an AP: S =(n/2)[2a + (n- 1)d]
The sum of n terms is also equal to the formulawhere l is the last term.
T_n = S_n – S_n-1 , where T_n = n^th term
When three quantities are in AP, the middle one is called as the arithmetic mean of the other two. If a, b and c are three terms in AP then b = (a+c)/2

Geometric Progression

A geometric progression is a sequence in which each term is derived by multiplying or dividing the preceding term by a fixed number called the common ratio. For example, the sequence 4, -2, 1, – 1/2,…. is a Geometric Progression (GP) for which – 1/2 is the common ratio.
The general form of a GP is a, ar, ar², ar³ and so on.
The nth term of a GP series is T_n = ar^n-1, where a = first term and r = common ratio = T_n/T_n-1) .
The formula applied to calculate sum of first n terms of a GP:
When three quantities are in GP, the middle one is called as the geometric mean of the other two. If a, b and c are three quantities in GP and b is the geometric mean of a and c i.e. b =√ac
The sum of infinite terms of a GP series S_∞= a/(1-r) where 0< r<1.
If a is the first term, r is the common ratio of a finite G.P. consisting of m terms, then the nth term from the end will be = ar^m-n.
The nth term from the end of the G.P. with the last term l and common ratio r is l/(r^(n-1)) .

Harmonic Progression

A series of terms is known as a HP series when their reciprocals are in arithmetic progression.
Example: 1/a, 1/(a+d), 1/(a+2d), and so on are in HP because a, a + d, a + 2d are in AP.
The n^th term of a HP series is T_n =1/ [a + (n -1) d].
In order to solve a problem on Harmonic Progression, one should make the corresponding AP series and then solve the problem.
nth term of H.P. = 1/(nth term of corresponding A.P.)
If three terms a, b, c are in HP, then b =2ac/(a+c).

Some General Series

Sum of first n natural numbers =
Sum of squares of first n natural numbers =
Sum of cubes of first n natural numbers =

About Post Author

Rv. Cooper

Rv. Cooper is an experienced news writer known for their accuracy, timeliness, and compelling storytelling. They have covered a range of beats and their work has been featured in prominent publications.

rahul100111@gmail.com